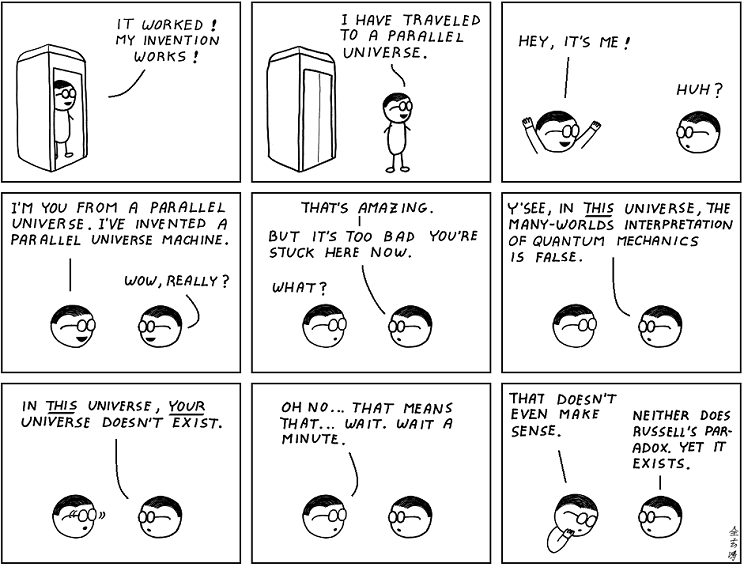
Roughly — very roughly — the many-worlds interpretation of quantum mechanics says that any given measurement of reality depends on the observer doing the measuring. And if no observer measures a reality, that reality just sort of disappears or collapses or something.
The many-worlds interpretation says wait! maybe the unobserved measurement really just goes off and exists in its own reality, its own world. And that all worlds with all possibly observed measurements all kind of co-exist simultaneously. AG is mildly obsessed by this stuff.
Anyway, this time AG has a guy in one world meeting himself in another but — well, you see where it goes. And I looked up Russell’s Paradox for you, and apparently it has to do with statements that aren’t lies and aren’t truths and are simply inconsistent. But on the whole, you’re on your own here. I’m not cut out for these kinds of shenanigans.
__________
Isn’t this the whole point of Godel’s Incompleteness Theorem? That as soon as any logical framework permits recursion, then it becomes possible to create meaningless (in logical terms rather than, say ‘political election promises’) statements which are correct within that framework.
The Epimenides paradox being one of the early formulations.
If I knew what “incompleteness,” “recursion,” and “Epimenides” meant, especially “recursion,” I’m pretty sure I’d agree.
Epimenides (of Crete) was a (possibly mythological) Greek philosopher of the Orphic tradition. He is most notable, apart from the paradox himself, for causing St Paul to have a complete breakdown of logic in the Epistle to Titus.
Incompleteness – the common English meaning. In this case, Godel’s statement is that once you allow a logical framework to become complex, it cannot be complete. This was a bit of a pain for Bertrand Russell who, along with Whitehead, had published “Principia Mathematica” in 1910, trying to establish a complete and consistent basis for all of maths, including the complicated stuff.
Recursion – actually, the Wikipedia definition is fine. “Self-referential” is another way of looking at it.
Aha! “a recursive definition . . . is used to define an object in terms of itself.” Thank you, Surreptitious Evil. I see why you mentioned politics. On the whole, I’m with St. Paul.